HSC EV Higher Mathematics 2nd Paper 6th Chapter Note

HSC EV Higher Mathematics 2nd Paper 6th Chapter Note. Conics. In mathematics, a conic section (or simply conic) is a curve obtained as the intersection of the surface of a cone with a plane. The three types of the conic section are the hyperbola, the parabola, and the ellipse. The circle is a special case of the ellipse and is of sufficient interest in its own right that it was sometimes called the fourth type of conic section. The conic sections have been studied by the ancient Greek mathematicians with this work culminating around 200 BC when Apollonius of Perga undertook a systematic study of their properties.

HSC EV Higher Mathematics 2nd Paper 6th Chapter Note. Conics

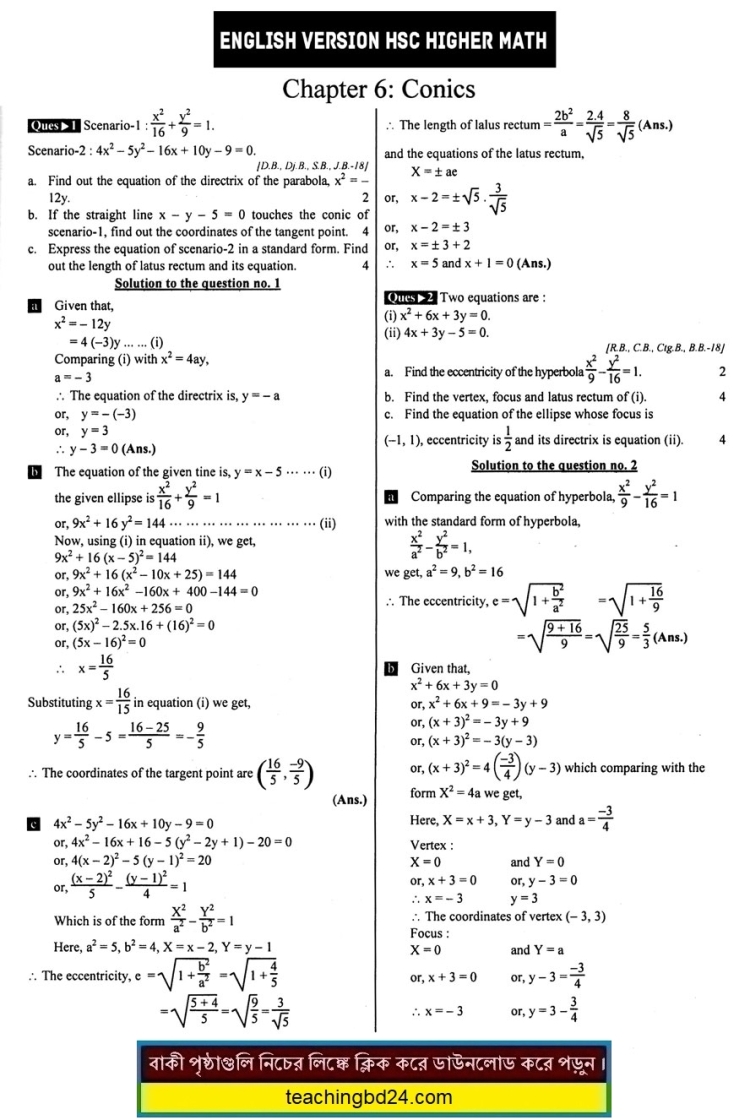
The conic sections of the Euclidean plane have various distinguishing properties. Many of these have been used as the basis for a definition of the conic sections. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a focus, and some particular line, called a directrix, are in a fixed ratio, called the eccentricity. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of degree 2; that is, as the set of points whose coordinates satisfy a quadratic equation in two variables. This equation may be written in matrix form, and some geometric properties can be studied as algebraic conditions.

The complex number system can be defined as the algebraic extension of the ordinary real numbers by an imaginary number i. This means that complex numbers can be added, subtracted, and multiplied, as polynomials in the variable I, with the rule i2 = −1 imposed. Furthermore, complex numbers can also be divided by nonzero complex numbers. Overall, the complex number system is a field.

Most importantly the complex numbers give rise to the fundamental theorem of algebra: every non-constant polynomial equation with complex coefficients has a complex solution. This property is true of the complex numbers, but not the reals. The 16th-century Italian mathematician Gerolamo Cardano is credited with introducing complex numbers in his attempts to find solutions to cubic equations.

Determinants occur throughout mathematics. For example, a matrix is often used to represent the coefficients in a system of linear equations, and the determinant can be used to solve those equations, although more efficient techniques are actually used, some of which are determinant-revealing and consist of computationally effective ways of computing the determinant itself. The use of determinants in calculus includes the Jacobian determinant in the change of variables rule for integrals of functions of several variables. Determinants are also used to define the characteristic polynomial of a matrix, which is essential for eigenvalue problems in linear algebra. In analytic geometry, determinants express the signed n-dimensional volumes of n-dimensional paralleled. Sometimes, determinants are used merely as a compact notation for expressions that would otherwise be unwieldy to write down. When the entries of the matrix are taken from a field (like the real or complex numbers), it can be proven that any matrix has a unique inverse if and only if its determinant is nonzero. Various other theorems can be proved as well, including that the determinant of a product of matrices is always equal to the product of determinants; and, the determinant of a Hermitian matrix is always real.

Is actually a polynomial because it’s possible to simplify this to 3x + 1 –which of course satisfies the requirements of a polynomial. (Remember the definition states that the expression ‘can’ be expressed using addition, subtraction, multiplication. So, if it’s possible to simplify an expression into a form that uses only those operations and whose exponents are all positive integers…then you do indeed have a polynomial equation)

teachingbd24.com is such a website where you would get all kinds of necessary information regarding educational notes, suggestions and questions’ patterns of school, college, and madrasahs. Particularly you will get here special notes of physics that will be immensely useful to both students and teachers. The builder of the website is Mr. Md. Shah Jamal Who has been serving for 30 years as an Asst. Professor of BAF Shaheen College Dhaka. He expects that this website will meet up all the needs of Bengali version learners /students. He has requested both concerned students and teachers to spread this website home and abroad.

English Version HSC Higher Math Note